how to find instantaneous rate of change

You can find the instantaneous rate of change of a function at a point by finding the derivative of that function and plugging in the #x#-value of the point.

Instantaneous rate of change of a function is represented by the slope of the line, it tells you by how much the function is increasing or decreasing as the #x#-values change.

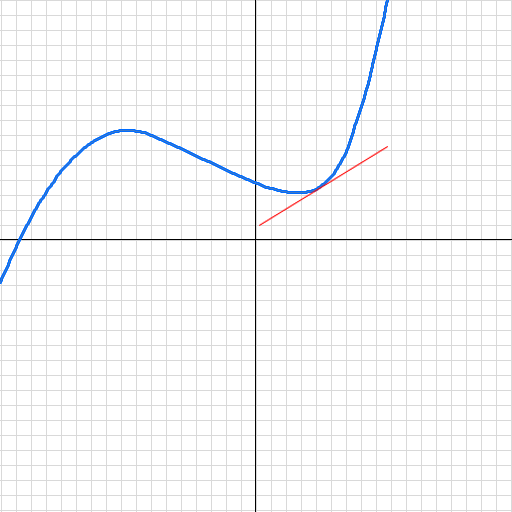
enter image source here
Figure 1. Slope of a line

In this image, you can see how the blue function can have its instantaneous rate of change represented by a red line tangent to the curve. To find the slope of this line, you must first find the derivative of the function.

Ex: #2x^2+4 , (1,6)#

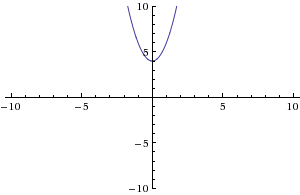
enter image source here
credit: www.wolframalpha.com

Using the power rule for derivatives, we end up with #4x# as the derivative. Plugging in our point's #x#-value, we have:

#4(1) = 4#

This tells us that the slope of our original function at #(1,6)# is #4#, which also represents the instantaneous rate of change at that point.

If we also wanted to find the equation of the line that is tangent to the curve at the point, which is necessary for certain applications of derivatives, we can use the Point-Slope Form:

#y-y_1=m(x-x_1)#

with #m# = slope of the line.

Plugging in our #x#,#y#, and slope value, we have:

#y-6=4(x-1)#

Which simplifies to

#y=4x+2#

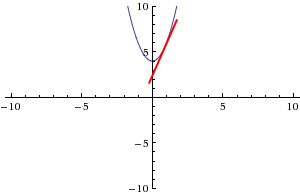
Problems involving "instantaneous rate of change" of a function require you to use the derivative, though it could be disguised in a way you may not be familiar with, such as the velocity of an object after a certain amount of time. Practicing similar rate of change problems will help you get a feel for the practical uses of derivatives.

how to find instantaneous rate of change

Source: https://socratic.org/questions/how-do-you-find-the-instantaneous-rate-of-change-of-a-function-at-a-point#:~:text=You%20can%20find%20the%20instantaneous,x%20%2Dvalue%20of%20the%20point.

Posted by: lopezovelly59.blogspot.com